Answer:
They will meet at a distance 9 miles from Karen's house.
Explanation:
Let the distance covered by Josh be
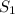 and the distance covered by Karen be
and the distance covered by Karen be
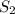
The distance between Josh and Karen's houses is 20 miles. That is, the total distance both of them will cover is 20 miles.
Hence,
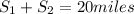
From the question, if Josh takes a 10 minute break after he starts while Karen rides the whole time, this means
If Karen rides for a total of
 mins, then Josh would spend
mins, then Josh would spend
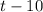 mins for the journey
mins for the journey
Also, Josh rides at an average speed of 15 miles per hour
and Karen rides at an average speed of 10 miles per hour
Now, for Josh
Distance =
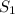
Speed = 15 mile/hour (Covert to mile/minute); (NOTE: 1 hour = 60 mins)
Speed= 0.25 mile/min
Time spent =
 mins
mins
From

Distance = Speed × Time
Distance = 0.25 mile/min × (t-10) min
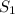 = 0.25(t-10) mile
= 0.25(t-10) mile
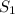 = 0.25t - 2.5
= 0.25t - 2.5
t = (
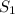 + 2.5) / 0.25 ....... (1)
+ 2.5) / 0.25 ....... (1)
For Karen,
Distance =
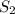
Speed = 10 mile/hour (Covert to mile/minute);
Speed=
 mile/min
mile/min
Time spent = t

Distance = Speed × Time
Then,
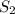 =
=
 mile/min × t min
mile/min × t min
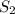 =
=
 mile
mile
t = 6
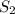 ......... (2)
......... (2)
Equating equations (1) and (2), we get
(
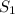 + 2.5) / 0.25 = 6
+ 2.5) / 0.25 = 6
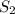
Then,
(
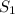 + 2.5) = 1.5
+ 2.5) = 1.5
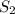
Recall that
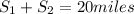
Then,
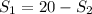
∴
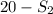 + 2.5 = 1.5
+ 2.5 = 1.5
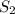
2.5
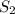 = 22.5
= 22.5
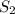 = 22.5 / 2.5
= 22.5 / 2.5
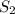 = 9 miles
= 9 miles
This means Karen covers a total distance of 9 miles
Hence, they will meet at a distance 9 miles from Karen's house.