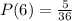Question

Consider a problem where we are rolling 2 dices where each dice has 6 faces numbered from 1 to 6.Answer the following questions:(1) What is the sample space?(2) If the event we are interested in is the sum being 10, what would be the probability of observingsuch an event?(3) If the event we are interested in is the sum being 6, what would be the probability of observingsuch an event?

Answer 1

1. See Explanation for Sample Space

2.
`P(10) = (1)/(12)`

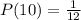
3.
`P(6) = (5)/(36)`

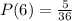
Explanation:

Given

Roll of Two die

Solving (1): The Sample Space

Represent the first dice with S1 and the second with S2

`S_1 = \{1,2,3,4,5,6\}`

`S_2 = \{1,2,3,4,5,6\}`

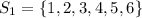
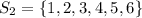
The sample space of both die is:

`S = (1,1)(1,2)(1,3)(1,4)(1,5)(1,6)(2,1)(2,2)(2,3)(2,4)(2,5)(2,6)(3,1)(3,2)(3,3)(3,4)(3,5)(3,6)`
`(4,1)(4,2)(4,3)(4,4)(4,5)(4,6)(55,1)(5,2)(5,3)(5,4)(5,5)(5,6)(6,1)(6,2)(6,3)(6,4)(6,5)(6,6)}\}`

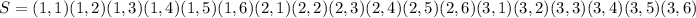
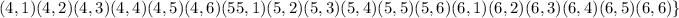
Solving (2): Probability of Sum being 10

First, we need to add the outcome of each roll in (1) above:

`S = \{2,3,4,5,6,7, 3,4,5,6,7,8, 4,5,6,7,8,9, 5,6,7,8,9,10, 6,7,8,9,10,11 7,8,9,10,11,12\}`
`n(S) = 36`

`n(10) = 3`

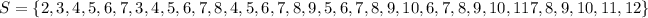
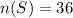
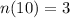
The required probability is:

`P(10)= (3)/(36)`

`P(10) = (1)/(12)`

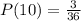
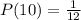
Solving (3): Probability of Sum being 6

`n(S) = 36`

`n(6) = 5`

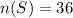
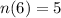
The required probability is:

`P(6) = (5)/(36)`