Answer:
24 m
Explanation:
The lenght of fencing needed is the sum of all sides the triangular garden represented on the coordinate grid.
The lenght of the triangular garden = 16 - 10 = 6 m
Lenght of the height = 8 - 0 = 8 m
Lenght of the hypotenuse can be calculated using coordinates of the two vertices of the ∆ that forms the hypotenuse lenght and also using the distance formula.
Coordinates of the two vertices = (10, 0) and (16, 8).

Let,
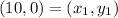
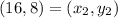
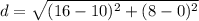

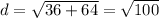
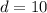
Length of fencing = 6 + 8 + 10 = 24 m