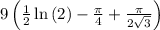Question

Why did I get this question wrong?

Answer 1

`9\left((1)/(2)\ln \left(2\right)-(\pi )/(4)+(\pi )/(2√(3))\right)`

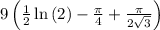
Explanation:

We are given the integral 9 arctan(1/x)dx on the interval x[ from 1 to √3 ].

Now let's say that u = arctan(1/x). The value of 'du' would be as follows:

du = - x / (1 + x²) * dx

If we apply integration by parts, v = 1, and of course u = arctan(1/x):

=> 9x arctan(1/x) − ∫ -9x dx / (x² + 1)

=> 9[x arctan(1/x) - ∫ - x / (1 + x²) * dx] on the interval x[ from 1 to √3 ]

Let's now simplify the expression ' ∫ - x / (1 + x²) * dx':

=> (Take the constant out, in this case constant = - 1), - ∫ x / (1 + x²) * dx

=> (Apply u-substitution, where u = 1 + x²), - ∫ 1/2u * du

=> (Take constant out again, in this case 1/2), - 1/2 ∫ 1/u * du

=> (Remember that 1/u * du = In( |u| )), - 1/2In( |u| )

=> (Substitute back 'u = 1 + x²), - 1/2In| 1 + x² |

So now we have the expression '9[x arctan(1/x) + 1/2In| 1 + x² |]' on the interval x[ from 1 to √3 ]. Let's further simplify this expression;

`9\left[x\arctan \left((1)/(x)\right)+(1)/(2)\ln \left|1+x^2\right|\right]^(√(3))_1\\\\=> 9\left[(1)/(2)\left(2x\arctan \left((1)/(x)\right)+\ln \left|1+x^2\right|\right)\right]^(√(3))_1`

Now computing the boundaries we have the following answer:

`9\left((1)/(2)\ln \left(2\right)-(\pi )/(4)+(\pi )/(2√(3))\right)`

Answer 2

Explanation:

∫ 9 arctan(1/x) dx

If u = 9 arctan(1/x), then:

du = 9 / (1 + (1/x)²) (-1/x²) dx

du = -9 dx (1/x²) / (1 + (1/x²))

du = -9 dx / (x² + 1)

If dv = dx, then v = x.

∫ u dv = uv − ∫ v du

= 9x arctan(1/x) − ∫ -9x dx / (x² + 1)

= 9x arctan(1/x) + 9/2 ∫ 2x dx / (x² + 1)

= 9x arctan(1/x) + 9/2 ln(x² + 1)

Evaluate from x=1 to x=√3.

[9√3 arctan(1/√3) + 9/2 ln(3 + 1)] − [9 arctan(1) + 9/2 ln(1 + 1)]

[9√3 (π/6) + 9/2 ln(4)] − [9 (π/4) + 9/2 ln(2)]

(3π√3)/2 + 9/2 ln(4) − (9π/4) − 9/2 ln(2)

(6π√3)/4 + 9 ln(2) − (9π/4) − 9/2 ln(2)

(6π√3 − 9π)/4 + 9/2 ln(2)