Answer:
3x -y = -4
Explanation:
You want the standard form equation of the line through (-1, 1) and (1, 7).
Development
The slope of a line is the same everywhere, so we can equate the slope for the given points to the slope for an arbitrary point:
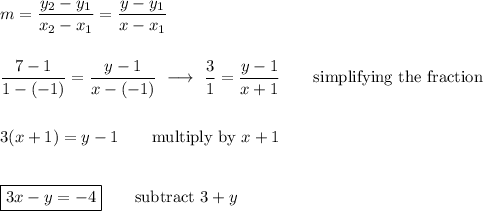
__
Additional comment
The standard form equation of a line is ax+by=c, where a>0 and a, b, c are mutually prime (have no common factors).
This development will work for any line with defined slope. It may be easier to remember than (y2-y1)(x-x1) -(x2 -x1)(y -y1) = 0, which is a variation that will always give a general form equation even for vertical lines. To turn general form to standard form, you can subtract the constant. In any event, the leading sign and common factor may need to be dealt with.
<95141404393>