Question

a) The radius of a circular field is 42 m. Find the perimeter of the field. Also find the length of wire required to fence it with 5 rounds ​

Answer 1

263.89

263.89*5=1319.45

Answer 2

We are given with the radius of the circular field,

`r = 42 \: m`

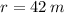
And, we have to find the perimeter of the field. Also, we have to find the length of the wire required to fence it with 5 rounds:

We know,

Perimeter of a circle = 2πr, where r is the radius of the circle, So let's find the perimeter by using this formula.

`perimeter = 2\pi r`

`perimeter = 2 * (22)/(7) * \: 42 m`

`perimeter = 2 * 22 * 6 \: m`

`perimeter = \boxed{264 \: m}`

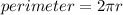
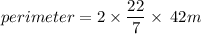
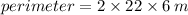
Now, finding the length of the wire which is equals to 5 × Perimeter of the circular field.

`length \: of \: wire = 264 \: m * 5`

`length \: of \: wire = \boxed{1320 \: m}`

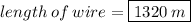
And we are done !!

#CarryOnLearning.

━━━━━━━━━━━━━━━━━━━━