Question

5x-2=3x+4
which one?
x=1
x=2/3
x=-3
x=3

Answer 1

x=3

Explanation:

Answer 2

`\boxed {\tt x=3}`

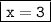
Explanation:

We are given the equation:

`5x-2=3x+4`

We want to solve for x, therefore we must isolate x on one side of the equation.

First, move all the constants to one side of the equation. 2 is being subtracted from 5x. The inverse of subtraction is addition. Add 2 to both sides.

`5x-2+2=3x+4+2`

`5x=3x+4+2`

`5x=3x+6`

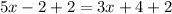
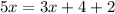
Next, move all the terms with variables to one side. 3x is being added to 6. The inverse of addition is subtraction. Subtract 3x from both sides.

`5x-3x=3x-3x+6`

`5x-3x=6`

`2x=6`

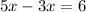
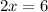
x is being multiplied by 2. The inverse of multiplication is division. Divide both sides of the equation by 2.

`(2x)/(2) =(6)/(2)`

`x=(6)/(2)`

`x=3`

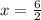
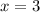
x is equal to 3 and the correct answer is D. x=3