Question

What is the range of the function below in set builder notation? y=|x-3|

Answer 1

Answer:
`\{y | \ y \in \mathbb{R}, \ y \ge 0\}`

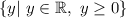
This translates to "y is any real number such that it is 0 or larger".

The reasoning is that the result of any absolute value function is either 0 or positive. In other words, we'll never get a negative result of an absolute value function. This is due to how absolute value represents distance. Negative distance does not make sense.

So if y = |x-3| then y = 0 is the smallest output possible. We could have any positive output we want.

In terms of a graph (see below), the V shape is at the lowest point (3,0). The y coordinate is all we care about in terms of finding the range. So we see the lowest y value is y = 0.