Answer:
Linear equation with a slope of 2 that goes through the point (3, 4) is
 .
.
Explanation:
From statement we know the slope of the line and a point contained in it. Using the slope-point equation of the line is the quickest approach to determine the appropriate equation, whose expression is:
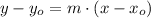
Where:
 - Slope, dimensionless.
- Slope, dimensionless.
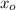 ,
,
 - Components of given point, dimensionless.
- Components of given point, dimensionless.
 ,
,
 - Independent and dependent variable, dimensionless.
- Independent and dependent variable, dimensionless.
If we know that
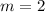 ,
,
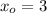 and
and
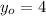 , the linear equation is found after algebraic handling:
, the linear equation is found after algebraic handling:
1)
 Given
Given
2)
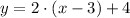 Compatibility with Addition/Existence of Additive Inverse/Modulative Property
Compatibility with Addition/Existence of Additive Inverse/Modulative Property
3)
 Distributive Property/
Distributive Property/
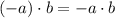 /Definition of sum/Result
/Definition of sum/Result
Linear equation with a slope of 2 that goes through the point (3, 4) is
 .
.