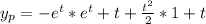Question

Find the particular solution to ty''-(1+t)y'+y=t^2e^t.

Answer 1

Complete Question

Find a particular solution to
`ty''-(1+t)y'+y=t^2e^t , t > 0`

`y_1 = 1 + t , \ y_2 = e^t`

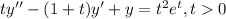
Answer:

The solution is
`y_p = -e^(2t) + t + (3t^2)/(2) + (t^3)/(2)`

Explanation:

Now the given equation is
`ty''-(1+t)y'+y=t^2e^t , t > 0`

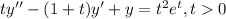
dividing through by t

We have

`y'' - (1 + t )/(t)y' + (1)/(t) y = te^t \\\\ let's\ call\ it \ g(t)`

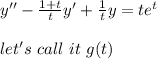
Now for the given initial value we can generate our Wronskian as follows

`W(y_1 ,y_2) = | \left y_1} \atop {y_1'}} \right. \left y_2} \atop {y_2'}} \right | = | \left {(1 + t)} \atop {1}} \right. \left e^t} \atop {e^t}} \right | = (1 + t )e^t - e^t = te^t`

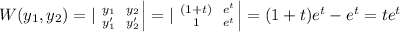
Now applying method of variation of parameters to obtain the particular solution

So here we assume that

`y_p = v_1 y_1 + v_2 y_2`

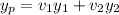
So

`v_1 = - \int\limits {(y_2 (t) g(t))/(W(t)) } \, dt = - \int\limits {(e^t te^t)/(te^t) } = - \int\limits { e^t} } = -e^t`

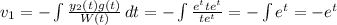
And

`v_2 = - \int\limits {(y_1 (t) g(t))/(W(t)) } \, dt = - \int\limits {((1 + t) te^t)/(te^t) } = - \int\limits (1 + t) = t + (t^2)/(2)`

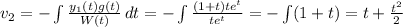
So

`y_p = -e^t * e^t + t + (t^2)/(2) * 1 + t`

`y_p  =  -e^(2t)   +  t +   (3t^2)/(2) + (t^3)/(2)`