Question

Find tan(13pi/4)-cos(pi/3)

Answer 1

Answer:
`tan((13\pi )/(4))-cos((\pi )/(3))=(1)/(2)`

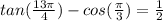
Explanation:

To find
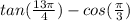`tan((13\pi )/(4))-cos((\pi )/(3))`, we first need to understand the unit circle. Let's find the value of tan and cos before we subtract them.

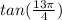`tan((13\pi )/(4) )` is the same as
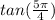`tan((5\pi )/(4) )`. The point for
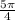`(5\pi )/(4)` is (-0.5,-0.5). Tangent is
`(sin)/(cos)`. Since sin and cos are both -0.5, we can divide them.

`(-0.5)/(-0.5) =1`

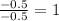
Now, we know that
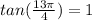`tan((13\pi )/(4) )=1`. All we have to do is find
`cos((\pi )/(3))`.

`cos((\pi )/(3))=(1)/(2)`

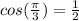
Now that we know the values of both, we can directly subtract them.

1-0.5=0.5

Therefore,
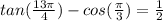`tan((13\pi )/(4))-cos((\pi )/(3))=(1)/(2)`.