Answer:
V = 288.5

Explanation:
To find the volume of a cone, use this equation:

r = radius
h = height
We aren't given the height of the cone, just the length of the side. To find the height use the Pythagorean Theorem:
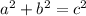
The value of 12.1 yds is the hypotenuse and the radius is the value of one of the legs. So, set it up like this:
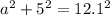
Now, solve:
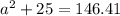 Distribute the square
Distribute the square
 Subtract 25 from 146.41
Subtract 25 from 146.41

a = 11.01862
So, the height is equal to 11.01862 yds.
Now, we can plug everything into the volume equation:

Simplify (use calculator):
V = 288.5

So, the volume of the cone is equal to 288.5
