How do i do this question? i can factor it by an method

Question

asked Feb 22, 2023 199k views

1 Answer

Jacob Petersen · Answer 1 · 2023-02-27T21:38:45+0000

Answer:

The x values are the same, so leave these be. They do not need to be factored. We need to find a way to factor y^4 and y^2

To do this, just factor using the formula for the difference between two squares

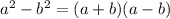
$a^2-b^2 = (a+b)(a-b)\\$

Substitute. A = y, b = 1

y^2(y+1)(y-1)

Since x^3 is the same, insert this before y^2

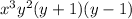
$x^3y^2(y+1)(y-1)\\$

This is the factored form.

Explanation: