Question

Find the coordinates of the midpoint of AS if A(-4, 7) and S(5,3).

Answer 1

`\boxed{ \bold{ \huge{ \boxed{ \sf{(0.5 \: , \: 5)}}}}}`

Explanation:

Let A ( -4 , 7 ) be ( x₁ , y₁ ) and S ( 5 , 3 ) be ( x₂ , y₂ )

Finding the midpoint

`\boxed{ \sf{midpoint = ( (x1 + x2)/(2) \: , \: (y1 + y2)/(2))}}`

`\longrightarrow{ \sf{( ( - 4 + 5)/(2) \: , \: (7 + 3)/(2)) }}`

`\longrightarrow{ \sf{( (1)/(2) \:, \: (10)/(2) }})`

`\longrightarrow{ \sf{( 0.5 \: , \: 5}})`

Hope I helped!

Best regards! :D

Answer 2

The answer is

`( (1)/(2) \: , \: 5)`

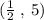
Explanation:

The midpoint M of two endpoints of a line segment can be found by using the formula

`M = ( (x1 + x2)/(2) , \: (y1 + y2)/(2) )`

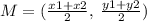
where

(x1 , y1) and (x2 , y2) are the points

From the question the points are

A(-4, 7) and S(5,3)

The midpoint is

`M = ( ( - 4 + 5)/(2) , \: (7 + 3)/(2) ) \\ = ( (1)/(2) , \: (10)/(2) )`

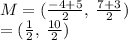
We have the final answer as

`( (1)/(2) \: , \: 5)`

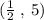
Hope this helps you