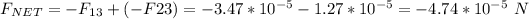Question

What is the net force exerted by these two charges on a third charge q3 = 54.5 nC placed between nC and nC at x3 = -1.145 m ? Your answer may be positive or negative, depending on the direction of the force.

Answer 1

`-4.74*10^(-5)\ N`

Step-by-step explanation:

Consider two point charges located on the x axis: one charge, q1 = -19.5 nC , is located at x1 = -1.670 m ; the second charge, q2 = 34.0 nC , is at the origin (x=0).

Given that:

q1 = -19.5 nC , x1 = -1.670 m, q2 = 34.0 nC , x2=0, q3 = 54.5 nC, x3 = -1.145 m

From Coloumbs law, the force between two charges is given by the formula:

`F=k(q_1q_2)/(d^2)\\ \\Where\ k=(1)/(4\pi \epsilon_o)=9*10^9\ Nm^2/C^2 ,\epsilon_o=8.854*10^(-12)\ C^2/Nm^2\\d=distance\ between\ charges`

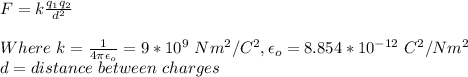
Hence the force of attraction of q1 on q3 is given by:

`F_(13)=k(|q_1q_3|)/((x_3-x_1)^2)=9*10^9((|-19.5*10^(-9)*54.5*10^(-9)|)/((-1.145-(-1.670))^2) )=3.47*10^(-5)\ N`

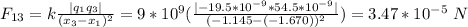
Hence the force of attraction of q2 on q3 is given by:

`F_(23)=k(|q_2q_3|)/((x_3-x_2)^2)=9*10^9((|34*10^(-9)*54.5*10^(-9)|)/((-1.145-0)^2) )=1.27*10^(-5)\ N`

The force of attraction of q1 on q3 is in the negative direction and force of attraction of q2 on q3 is in the negative direction. Hence the net force is:

`F_(NET)=-F_(13)+(-F{23})=-3.47*10^(-5)-1.27*10^(-5)= -4.74*10^(-5)\ N`