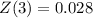Answer:
for n = 3
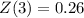
for n = 10
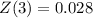
Explanation:
from the question we are that
The number of applicant is n
The number of people on the committee is
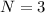
Generally given that the applicant ranking is random the probability of a candidate being accepted is mathematically represented as

Now for n = 3
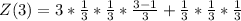
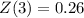
Now for n = 10