Question

Find the equation of the line. Use exact numbers.

Answer 1

`y=-(3)/(2)x+3`

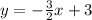
Explanation:

We can find an equation for the line by using the slope of the line and the y-intercept.

We can see that the y-intercept of of the line is 3.

To find the slope of the line, we can use the slope formula. The slope formula is:

`m=(y_2-y_1)/(x_2-x_1)`

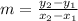
where (x₁, y₁) and (x₂, y₂) are points on the line. To find the slope of the line, I will use the x-intercept, which is (2, 0), for (x₂, y₂) and y-intercept, which is (0, 3), for (x₁, y₁).

`m=(0-3)/(2-0)=(-3)/(2)=-(3)/(2)`

So now we know the y-intercept and the slope, so lets plug it into the slope-intercept form equation. This gives us:

`y=-(3)/(2)x+3`

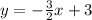
So the equation of the line would be
`y=-(3)/(2)x+3`.

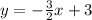I hope you find my answer helpful.

Answer 2

[see below]

Explanation:

Using two points on the line:

(0,3) (2,0)

Find the slope of the line:

`m=(rise)/(run)=(0-3)/(2-0)=(-3)/(2)=-(3)/(2)`

The slope of the line is
`-(3)/(2)`.

The y-intercept is (0,3).

The line equation should be:
`y=-(3)/(2)x+3`.

Hope this helps.