Question

A solution is made containing 14.6g of CH3OH in 185g H2O.1. Calculate the mole fraction of CH3OH.2. Calculate the mass percent of CH3OH.3. Calculate the molality of CH3OH.

Answer 1

*
`x_(CH_3OH)=0.0425`

*
`\%m/m_(CH_3OH)=7.31\%`

*
`m=2.46m`

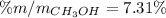
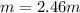
Step-by-step explanation:

Hello,

In this case, for the mole fraction of methanol we use the formula:

`x_(CH_3OH)=(n_(CH_3OH))/(n_(CH_3OH)+n_(water))`

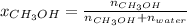
Thus, we compute the moles of both water (molar mass 18 g/mol) and methanol (molar mass 32 g/mol):

`n_(CH_3OH)}=14.6g*(1mol)/(32g)=0.456molCH_3OH \\\\n_(water)}=185g*(1mol)/(18g)=10.3molH_2O`

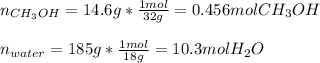
Hence, mole fraction is:

`x_(CH_3OH)=(0.456mol)/(0.456mol+10.3mol)\\\\x_(CH_3OH)=0.0425`

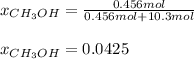
Next, mass percent is:

`\%m/m_(CH_3OH)=(m_(CH_3OH))/(m_(CH_3OH)+m_(water))*100\%\\\\\%m/m_(CH_3OH)=(14.6g)/(14.6g+185g)*100\%\\\\\%m/m_(CH_3OH)=7.31\%`

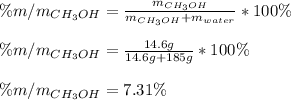
And the molality, considering the mass of water in kg (0.185 kg):

`m=(n_(CH_3OH))/(m_(water)) =(0.456mol)/(0.185kg)\\ \\m=2.46m`

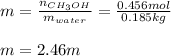
Regards.