Question

PLEASE HELP ME WITH QUESTION 7
SHOW YOUR WORK

Answer 1

(-2, 1) and (6, 1)

Explanation:

The standard form equations for a hyperbola are ...

`((x-h)^2)/(a^2)-((y-k)^2)/(b^2) = 1 \\\\((y-k)^2)/(a^2)-((x-h)^2)/(b^2) = 1`

The first form opens horizontally; the second opens vertically. Further, the center-focus distance 'c' is given by ...

`c^2 = a^2 +b^2 \qquad\text{$c$ = distance from center to focus}`

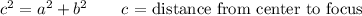
The attached figure illustrates the relation between the various parameters and the features of the hyperbola.

__

7.

Using the above information and the information in the first attachment, we find ...

(h, k) = (2, 1)

a = 4, b = 2

The vertices are (h±a, k), so are (2±4, 1) = (-2, 1) and (6, 1).

__

The second attachment illustrates the hyperbola and its vertices.