Answer:
47.
 or
or

50.
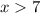 or
or
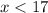
53.
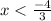 or
or
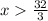
56.
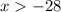 or
or
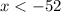
Explanation:
47.

-There are two equations:
Equation 1:
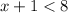
or
Equation 2:
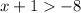
-Solving equation 1:
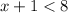
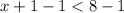

-Solving equation 2:
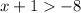
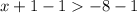

Answers:
 or
or

50.
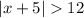
-There are two equations:
Equation 1:
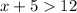
or
Equation 2:
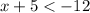
-Solving equation 1:
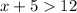
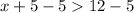
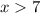
-Solving equation 2:
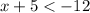

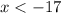
-Answers:
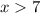 or
or
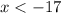
53.
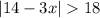
-There are two equations:
Equation 1:
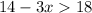
or
Equations 2:
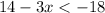
-Solving equation 1:
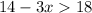
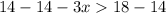
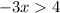
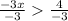
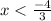 (Inequality sign changed, because of dividing by a negative number)
(Inequality sign changed, because of dividing by a negative number)
-Solve equation 2:
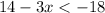
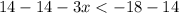
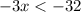
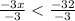
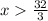 (Inequality sign changed, because of dividing by a negative number)
(Inequality sign changed, because of dividing by a negative number)
-Answers:
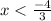 or
or
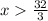
56.
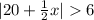
-Switch the equation:
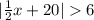
-There are two equations:
Equations 1:
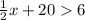
or
Equation 2:
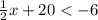
-Solving equation 1:
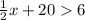
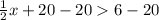
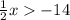
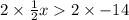
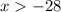
-Solving equation 2:
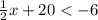
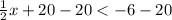
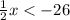
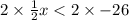
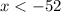
-Answers:
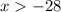 or
or
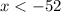
And were done.