Answer:
the length of the apothem is 8, option B
Explanation:
given:
a regular hexagon with side length 12 and radius 10.
find;
the length of the apothem
referring to the attached image,
to get the length of the apothem, use the Pythagorean theorem.
a² + b² = c²
let a = 6, b = apothem, c = 10
plugin values into the formula
6² + b² = 10²
b² = 10² - 6²
b =

b =
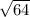
b = 8
therefore,
the length of the apothem is 8, option B