Answer:
70.6°
Explanation:
The angle between the 2 lines can be calculated using
tanΘ = |
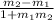 | ( Θ is the angle between the lines )
| ( Θ is the angle between the lines )
The equation of a line in slope- intercept form is
y = mx + c ( m is the slope and c the y- intercept )
Rearrange the 2 equations into this form to extract the slopes
3x - 4y + 5 = 0 ( subtract 3x + 5 from both sides )
- 4y = - 3x - 5 ( divide all terms by - 4 )
y =
 x +
x +
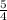 with m =
with m =
 ←
←
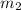
--------------------------------------------
2x + 3y - 1 = 0 ( subtract 2x - 1 from both sides )
3y = - 2x + 1 ( divide all terms by 3 )
y = -
 x +
x +
 with m = -
with m = -
 ←
←
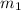
Note it does not matter which slope is labelled
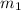 or
or
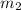
Thus
tan Θ = |
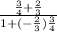
= |
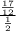 = |
= |
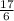 | =
| =
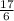 , then
, then
Θ =
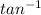 (
(
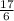 ) ≈ 70.6° ( to 1 dec. place )
) ≈ 70.6° ( to 1 dec. place )