Question

How to calculate y=6x^2+1

Answer 1

Hi there!

~

⇒ Graph :
`y=6x^2+1`

∴ Rewrite the equation in vertex form.

⇒
`y = 6 ( x + 0)^2 + 1`

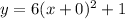
∴ Use the vertex form,
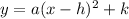`y = a(x - h)^2 + k` , to determine the values of
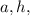`a , h ,` and
`k`

`a = 6 \\h = 0\\k = 1`

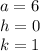
∴ Since the value of
`a` is positive, the parabola opens up.

⇵

∴ Find the vertex
`( h,k)`.

`(0,1)`

∴ Find
`p`, the distance from the vertex to the focus.

`(1)/(24)`

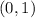
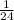
∴ Find the focus.

`( 0 , (25)/(24) )`

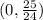
∴ Find the axis of symmetry by finding the line that passes through the vertex and the focus.

`x = 0`

∴ Find the directrix.

`y = (23)/(24)`

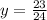
∴ Use the properties of the parabola to analyze and graph the parabola.

Direction: Opens Up

⇒ Vertex:
`( 0 , 1)`

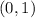
⇒ Focus:
`( 0 , (25)/(24) )`

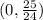
⇒ Axis of Symmetry:
`x = 0`

⇒ Directrix:
`y = (23)/(24)`

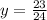
∴ Select a few
`x` values, and plug them into the equation to find the corresponding
`y` values. The
`x` values should be selected around the vertex.

`x : -2 , -1 , 0 , 1 , 2 \\y : 25 , 7 , 1 , 7 , 25`

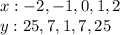
Hope this helped you!