Answer: X=15; 160°
Step-by-step explanation: Since these are opposite angles (or vertical angles), that means that both angles have the same measure. To find out what the angle measures are, set the two equations equal to each other.
Step 1: Set the equations equal to each other
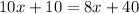
Step 2: Subtract 10 from both sides

Step 3: Subtract 8x from both sides
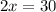
Step 4: Divide both sides by 2
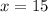
Step 5: Plug in the value of X to get the angle measures

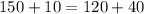
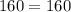
Since
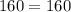 , we know that we solved the problem correctly, and the angle measure is 160°
, we know that we solved the problem correctly, and the angle measure is 160°