Question

11. A ranger in a lookout tower spots two fires on the campground below. Fire Als 75 meters east

and 40 meters south of the tower Fire B is 37 meters west and 64 meters south of the tower.
If there is a fire hydrant located three-fourths of the way from Fire A to Fire B, find the location
of the fire hydrant relative to the tower


Answer 1

The fire hydrant = 9 meters west and south of the tower = 58 m

Explanation:

The coordinate as the origin includes the Fire A and Fire B

Given that

Fire A is 75 meters east and south of the tower is 40 meters

So, it is presented (75,-40)

And, the fire B is 37 west and south of the tower is 64 meters

So, it is presented (-37,-64)

Now at a point O (x,y) divides AB a line segment as A(x_1, y_1) and B(x_2, y_2)

So,

`x = (n)/(n+m) (x_2 - x_1) + x_1\\\\y = (n)/(n+m) (y_2 - y_1) + y_1`

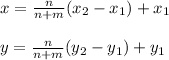
Now it divides in three-fourth

So,

`x = (n)/(n+m) (x_2 - x_1) + x_1\\\\= (3)/(4) (-37-75) + 75\\\\= (3)/(4) (-112) + 75\\\\= -84 + 75\\\\= -9`

`y = (n)/(n+m) (y_2 - y_1) + x_1\\\\= (3)/(4) (-64-(-40)) + (-40)\\\\= (3)/(4) (-18) -40\\\\= -18-40\\\\= -58`

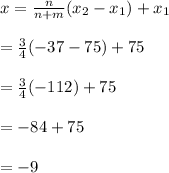
Hence, these two are to be considered