Answer:
The external pressure is p = -21.9 psf or p = -8.85 psf
Step-by-step explanation:
Given :
Velocity of wind, v = 120 mi / hr
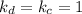 (wind direction factor)
(wind direction factor)
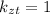 = topographical factor (for flat terrain)
= topographical factor (for flat terrain)
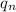 = velocity pressure at height h
= velocity pressure at height h
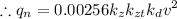

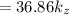
But for height h = 30 ft,
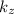 = 0.98 (from table)
= 0.98 (from table)
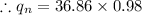
= 36.16
Now,
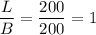 , so
, so
 (from table)
(from table)
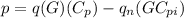
where, p = external pressure
G = 0.85 = gust factor (for typical rigid building)
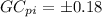 (internal pressure co efficient)
(internal pressure co efficient)
Therefore putting the values,
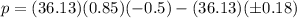
p = -21.9 psf or p = -8.85 psf