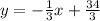Question

Write an equation of the line containing the given point and parallel to the given line. Express your answer in the form y=mx+b.

(7,9): x+3y=7

Answer 1

The equation of the parallel line is:

`y=-(1)/(3) x+(34)/(3)`

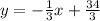
Explanation:

First express the given line in slope-intercept form:

`x+3\,y = 7\\3\,y = -x+7\\y=-(1)/(3) x+(7)/(3)`

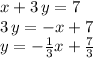
Since we want a line parallel to this, the new line must have the same slope (-1/3). We add to that the condition of the line going through the point (7, 9):

`y=-(1)/(3) x+b\\9=-(1)/(3) (7)+b\\9=-(7)/(3) +b\\b=9+(7)/(3) \\b=(34)/(3)`

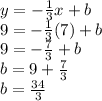
Therefore,the equation of the parallel line is:

`y=-(1)/(3) x+(34)/(3)`