Answer:
a
The null hypothesis is

The alternative hypothesis is

b
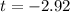
c
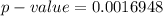
d
There no sufficient evidence to support the conclusion that the population mean sales prices for new one-family homes in the South is less expensive than the national mean of $181,900
Explanation:
From the question we are told that
The population mean is
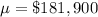
The sample size is

The sample mean is
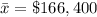
The sample standard deviation is
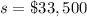
The null hypothesis is

The alternative hypothesis is

Generally the test statistics is mathematically represented as
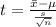
=>

=>
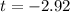
Generally the p-value is obtain from the z-table the value is
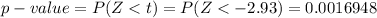
=>
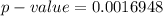
From the calculation we see that
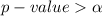 hence we fail to reject the null hypothesis
hence we fail to reject the null hypothesis
Thus there no sufficient evidence to support the conclusion that the population mean sales prices for new one-family homes in the South is less expensive than the national mean of $181,900