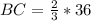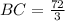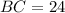Answer:
See Explanation
Explanation:
The question is incomplete as the number line is not attached.
However, the following will guide you through
Given
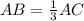
Required
Determine BC
First, we need to get the fraction of BC using the following
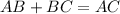
Substitute
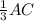 for AB
for AB
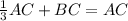
Make BC the subject of formula
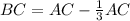
Take LCM
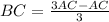
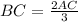
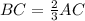
This implies that you multiply the length of AC by 2/3 to get BC
Take for instance, AC = 24
BC will be:
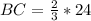
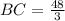
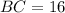
Or if AC = 12
BC will be:
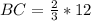
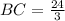
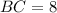
Or if AC = 36
BC will be: