Question

The curve given by x = sin(t) and y = sin(t + sin(t)) has two tangent lines at the point (x,y) = (0,0). List both of them in order of increasing slope. Your answers should be in the form of y = f(x) without t's.

Line with smaller slope: y(x):



Line with larger slope: y(x):

Answer 1

slope of the tangent

`(d y)/(d x) = \frac{cos(x+sin^(-1)(x) (1+\sqrt{1-x^(2)) } }{\sqrt{1-x^(2) } }`

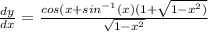
The function y = f(x)

y(x) = 2 x + C

Explanation:

Step(i):-

Given x= sin t ...(i)

Differentiating equation (i) with respective to 'x' , we get

`(d x)/(d t) = cost`

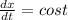
Given y = sin ( t + sin (t)) ...(ii)

Differentiating equation (ii) with respective to 'x' , we get

`(d y)/(d t) = cos (t + sin t ) (1 + cos t)`

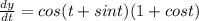
Step(ii):-

`(dy)/(dx) = ((dy)/(dt) )/((dx)/(dt) )`

`(d y)/(d x) = (cos(t+sin t) (1+cost))/(cost)`

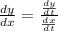
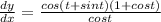
we know that

x = sin t

t = sin⁻¹ (x)

cost = √1 - sin²(t) = (√1-x²)

`(d y)/(d x) = \frac{cos(x+sin^(-1)(x) (1+\sqrt{1-x^(2)) } }{\sqrt{1-x^(2) } }`

`(d y)/(d x) = \frac{cos(x+sin^(-1)(x) (1+\sqrt{1-x^(2)) } }{\sqrt{1-x^(2) } }`

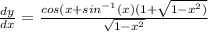
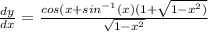
Put x =0 and y=0

`(d y)/(d x) = 2`

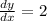
d y = 2 d x

Integrating with respective to 'x' , we get

y(x) = 2 x + C