Question

A rectangular wading pool measures 7 feet by 6 feet by 2.5 feet. When the pool is empty, it weighs 35 pounds. If water weighs 6.24 pounds per cubic foot, what is the total weight of the pool when it is full of water?

Answer 1

The total weight of the pool when it is full of water is 690.2 pounds.

Explanation:

We know that total weight of the wading pool (
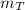`m_(T)`) is the sum of the weight of empty pool (
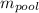`m_(pool)`) and the weight of water (
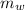`m_(w)`), all measured in pounds, that is:

`m_(T) = m_(pool) + m_(w)`

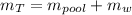
The weight of water can be determined by definition of density, as we know the geometry of the wading pool and density of water:

`m_(w)=\rho_(w)\cdot (w\cdot h\cdot l)`

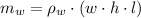
Where:

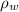`\rho_(w)` - Density, measured in pounds per cubic foot.

`w`,
`h`,
`l` - Width, height and length of the wading pool, measured in feet.

Now, we are noticed that
`\rho_(w) = 6.24\,(pd)/(ft^(3))`,
`w = 7\,ft`,
`h = 6\,ft`,
`l = 2.5\,ft` and
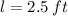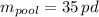`m_(pool) = 35\,pd` and the total weight of the pool when it is full of water is:

`m_(w) = \left(6.24\,(pd)/(ft^(3)) \right)\cdot (7\,ft)\cdot (6\,ft)\cdot (2.5\,ft)`

`m_(w) = 655.2\,pd`

`m_(T) = 35\,pd + 655.2\,pd`

`m_(T) = 690.2\,pd`

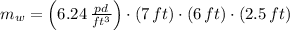
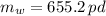
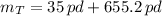
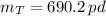
The total weight of the pool when it is full of water is 690.2 pounds.