Answer:
FG=30
Explanation:
Since we know that Point G is on the Segment FH, it doesn't really matter where G is, but we can know for certain that:
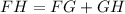
We are given that FH is 4x, GH is x, and FG is 2x+10. Substitute:

Solve for x. On the right, combine like terms:
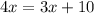
Subtract 3x from both sides:
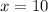
So, the value of x is 10.
To find the value of FG, substitute 10 into its x:
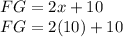
Multiply:
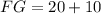
Add:
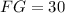
And we're done!