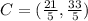Question

A university is building a new student center that is two- thirds the distance from the arts center to the residential complex. What are the coordinates of the new center? Explain.​

The points are (1, 9) and (9, 3)

Answer 1

`C = ((21)/(5),(33)/(5))`

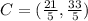
Explanation:

Given

Points: (1, 9) and (9, 3)

Ratio = 2/3

Required

Determine the coordinate of the center

Represent the ratio as ratio

`Ratio = 2:3`

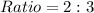
The new coordinate can be calculated using

`C = ((mx_2 + nx_1)/(n + m),(my_2 + ny_1)/(n + m))`

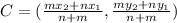
Where

`(x_1,y_1) = (1, 9)`

`(x_2, y_2) = (9, 3)`

`m:n = 2:3`

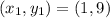
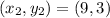
Substitute these values in the equation above

`C = ((2 * 9 + 3 * 1)/(3 + 2),(2 * 3 + 3 * 9)/(2 + 3))`

`C = ((18 + 3)/(5),(6 + 27)/(5))`

`C = ((21)/(5),(33)/(5))`

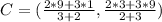
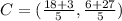
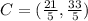
Hence;

The coordinates of the new center is
`C = ((21)/(5),(33)/(5))`