Question

The circles are identical. What is the circumference of each circle? Circle 1: radius of 2x; Circle 2: diameter of 2x+3. A. 3/2 B. 3 C. 1.5π D. 9π/4 E. 3π F. 6π

Answer 1

C=6π

Explanation:

It is given that, two circles are identical. The radius of first circle is 2x and the diameter of other circle is 2x+3.

If identical, it means that the radius of both circles are same i.e.

`2x=(2x+3)/(2)\ \ (\because\ r=(D)/(2), d=\text{diameter})\\\\4x=2x+3\\\\2x=3\\\\x=1.5`

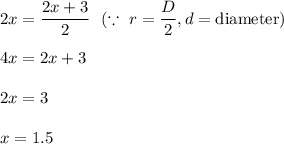
Radius of both circles is 2(1.5)=3

The circumference of a circle is given by :

`C=2\pi r\\\\C=2\pi (3)\\\\C=6\pi`

So, the circumference of each circle is 6π.