Question

Tickets for the homecoming dance cost $10 with ASB and $12 without ASB. If 920 tickets were sold and a total of $9,799 was collected, how many of each were sold?

Answer 1

Number of tickets with ASB is 620 and number of tickets without ASB is 300.

Explanation:

Let the number of tickets for the homecoming dance with ASB and without ASB are x and y respectively.

Total number of tickets = 920 tickets

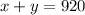`x+y=920` ...(1)

Tickets for the homecoming dance cost $10 with ASB and $12 without ASB. Total collected amount is $9,799

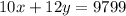`10x+12y=9799` ...(2)

From (1) and (2), we get

`10(920-y)+12y=9799`

`9200-10y+12y=9799`

`2y=9799-9200`

`2y=599`

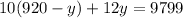
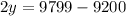
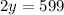
Divide both sides by 2.

`y=299.5`

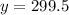
But number of tickets cannot be in decimal.

`y\approx 300`

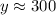
Put y=300 in (1).

`x+300=920`

`x=920-300`

`x=620`

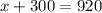
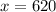
Therefore, number of tickets with ASB is 620 and number of tickets without ASB is 300.