Given :
Equation of line 1 , y = 2x .
A point (1 , 2) .
To Find :
The equation of the line L2 perpendicular to L1 passing through the point P = (1, 2) .
Solution :
Let , equation of line 2 is :
y = mx + c .....eq 1 ( here , m is slope and c is a constant )
Now , we know when two lines are perpendicular product of their slope is -1 .
Slope of given line is 2 .
Therefore ,
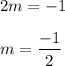
Now putting value of m in equation 1 , we get :
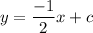
Now , it is given that this point (1,2) satisfy the above equation .
So ,
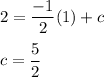
Putting value of c in above equation , we get :
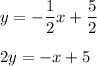
Therefore , the equation of the line L2 perpendicular to L1 passing through the point P = (1, 2) is 2y = -x +5 .
Hence , this is the required solution.