Question

What is the equation of the line parallel to 3x + 5y = 11 that passes through the point (15, 4)?

Answer 1

Answer:D

Step-by-step explanation:Edge

Answer 2

The answer is

`y = - (3)/(5) x + 13`

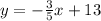
Explanation:

Equation of a line is y = mx + c

where

m is the slope

c is the y intercept

To find the equation of the parallel line we must first find the slope of the original line

The original line is 3x + 5y = 11

We must first write the equation in the general equation above

So we have

5y = - 3x + 11

Divide both sides by 5

`y = - ( 3)/(5) x + (11)/(5)`

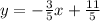
Comparing with the general equation above

Slope = - 3/5

Since the lines are parallel their slope are also the same

Slope of parallel line = - 3/5

So the equation of the line using point

(15, 4) and slope - 3/5 is

`y - 4 = - (3)/(5) (x - 15) \\y - 4 = - (3)/(5) x + 9 \\ y = - (3)/(5) x + 9 + 4`

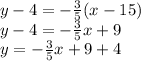
We have the final answer as

`y = - (3)/(5) x + 13`

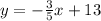
Hope this helps you