Answer:
14
Explanation:
From the given information:
suppose, Y is the number of times a coin is being flipped.
If the coin is flipped for the first time and we get H, then we have:
TTT =
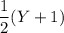
Afterward, if we get H, then we waste two times plus the probability of this event
 .
.
Therefore, we have :
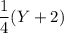
Afterward, if we get H, then we waste three times plus the probability of this event
 .
.
Therefore, we have :
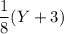
If we got T at the third time, then;
T =
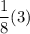
Thus, average number of headsthat you’ll see until gettingTTT can be expressed as:
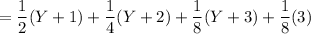
= 14