Question

Diego and Anya live 72 miles apart. They both meet at

their favorite restaurant, which is (16x – 3) miles from
Diego's house and (5x + 2) miles from Anya's house.
Diego argues that in a straight line distance, the restaurant
is halfway between his house and Anya's house. Is Diego
right? Justify your reasoning.

Answer 1

The restaurant is not at half way

Explanation:

Given

`Total\ Distance = 72`

`Diego = 16x - 3`

`Anya = 5x + 2`

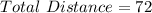
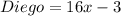
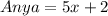
Required

Accept or reject Diego's claim

To do that, we need to determine the value of x by:

Diego + Anya =Total Distance

Substitute values for each parameter

`16x - 3 + 5x + 2 = 72`

Collect Like Terms

`16x + 5x = 72 + 3 - 2`

`21x = 73`

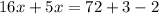
Divide both sides by 21

`x = (73)/(21)`

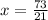
Substitute the value of x in Diego and Anya's distance

`Diego = 16x - 3`

`Diego = 16 * (73)/(21) - 3`

`Diego = (1168)/(21) - 3`

`Diego = (1168 - 63)/(21)`

`Diego = (1105)/(21)`

`Diego = 52(13)/(21)`

`Anya = 5x + 2`

`Anya = 5 * (73)/(21) + 2`

`Anya = (365)/(21) + 2`

`Anya = (365 + 42)/(21)`

`Anya = (407)/(21)`

`Anya = 19(8)/(21)`

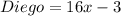
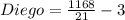
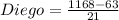
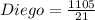
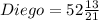
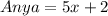
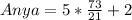
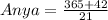
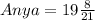
Since, both distances are not equal,

Then Diego's claim is false and incorrect