Question

Explain why a quadratic equation with a positive discriminant has two real solutions, a quadratic equation with a negative discriminant has no real solution, and a quadratic equation with a discriminant of zero has one real solution.

Answer 1

B (–4, –12)

Explanation:

If its even the right question

Answer 2

A quadratic equation can be written as:

a*x^2 + b*x + c = 0.

where a, b and c are real numbers.

The solutions of this equation can be found by the equation:

`x = (-b +- √(b^2 - 4*a*c) )/(2*a)`

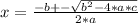
Where the determinant is D = b^2 - 4*a*c.

Now, if D>0

we have the square root of a positive number, which will be equal to a real number.

√D = R

then the solutions are:

`x = (-b +- R )/(2*a)`

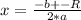
Where each sign of R is a different solution for the equation.

If D< 0, we have the square root of a negative number, then we have a complex component:

√D = i*R

`x = (-b +- C*i )/(2*a)`

We have two complex solutions.

If D = 0

√0 = 0

then:

`x = (-b +- 0)/(2*a) = (-b)/(2a)`

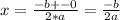
We have only one real solution (or two equal solutions, depending on how you see it)