Question

The graph of a function g is a

transformation of the graph of
f (x) = 9x + 5, where g(x) is 60% of
f(x). Write a rule for g

Answer 1

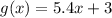`g(x)=5.4x+3`, graph of f(x) vertically compressed by factor 0.6 to get g(x).

Explanation:

The given function is

`f(x)=9x+5`

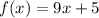
It is given that the graph of a function g is a transformation of the graph of

f (x), where g(x) is 60% of f(x). So, we need to write a rule for g.

`g(x)=60\%\text{ of }f(x)`

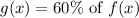
It means graph of f(x) vertically compressed by factor 0.6 to get g(x).

`g(x)=(60)/(100)(9x+5)`

`g(x)=0.6(9x+5)`

`g(x)=0.6(9x)+0.6(5)`

`g(x)=5.4x+3`

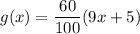
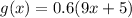
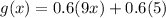
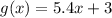
Therefore,
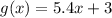`g(x)=5.4x+3`. Graph of f(x) vertically compressed by factor 0.6 to get g(x).