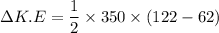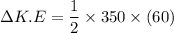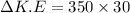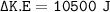Question

The change in kinetic energy (in joules) as a horse accelerates from canter to gallop is found using the expression 1/2 times 350 times (12 2 Minus 6 2

Answer 1

`\mathtt{\Delta K.E = 10500 \ J}`

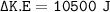
Explanation:

Given that:

the expression for the change in kinetic energy =
`(1)/(2) * 350 * ( 122 -62)`

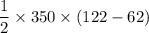
Recall that

Kinetic energy K.E =
`(1)/(2)mv^2`

where,

m = mass of the horse

v = velocity of the horse

The change in kinetic energy between two instant times can be expressed by the relation

`\Delta K.E = K.E_2 - K.E_1`

`\Delta K.E =(1)/(2)mv^2_2- (1)/(2)mv^2_1`

`\Delta K.E =(1)/(2)m(v^2_2-v^2_1)`

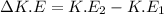
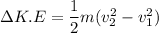
where;

m = 350

`v_2` = 122

`v_1`= 62

`\Delta K.E =(1)/(2) * 350 * (122-62)`

`\Delta K.E =(1)/(2) * 350 * (60)`

`\Delta K.E = 350 * 30`

`\mathtt{\Delta K.E = 10500 \ J}`