Question

Does the horizontal distance d travelled by the ball depend on the height of release? If it does depend on the height, what is the mathematical relationship between the variables

Answer 1

Step-by-step explanation:

Yes , the horizontal distance travelled by the ball will depend upon the height of release .

When a ball is thrown at some angle from a height , it has two components , the vertical component and horizontal component . The ball goes in horizontal direction due to its horizontal component . Its vertical component has no role to play . But the horizontal range covered by the body thrown

depends upon the duration of time in which it remains in air . The longer it remains in air , the greater distance it can cover horizontally .

Horizontal distance covered = t x horizontal velocity

If V be the velocity of throw and Vx be its horizontal component

Horizontal distance covered = t x Vx

Now t depends upon the height . If height rises , time of fall will increase so horizontal distance covered will increase .

If h be the height from which the body is thrown , Vy be the vertical upward component of initial velocity

from the relation

s = ut + 1/2 at²

h = - Vy t + 1/2 at²

As h increases , t will increase and therefore horizontal distance covered will increase. If the ball has only horizontal velocity initially , Vy = 0

h = 1/2 gt²

`t = \sqrt{(2h)/(g) }`

Horizontal distance covered = t x Vx

=
`\sqrt{(2h)/(g) } * V_x`

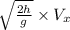
From this expression also

Horizontal distance covered is proportional to
`√(h)` .