Answer:
The volume of the tumor experimented a decrease of 54.34 percent.
Explanation:
Let suppose that tumor has an spherical geometry, whose volume (
 ) is calculated by:
) is calculated by:
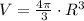
Where
 is the radius of the tumor.
is the radius of the tumor.
The percentage decrease in the volume of the tumor (
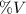 ) is expressed by:
) is expressed by:
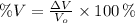
Where:
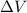 - Absolute decrease in the volume of the tumor.
- Absolute decrease in the volume of the tumor.
 - Initial volume of the tumor.
- Initial volume of the tumor.
The absolute decrease in the volume of the tumor is:
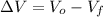
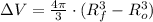
The percentage decrease is finally simplified:
![\%V = \left[1-\left((R_(f))/(R_(o))\right)^(3) \right]* 100\,\%](https://img.qammunity.org/2021/formulas/mathematics/high-school/kie5vkvf8coloal5dyfidyjzft9r2kbpot.png)
Given that
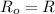 and
and
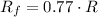 , the percentage decrease in the volume of tumor is:
, the percentage decrease in the volume of tumor is:
![\%V = \left[1-\left((0.77\cdot R)/(R)\right)^(3) \right]* 100\,\%](https://img.qammunity.org/2021/formulas/mathematics/high-school/su8nwff85vukeyd2dxevqt5yg5ryy5bx46.png)
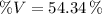
The volume of the tumor experimented a decrease of 54.34 percent.