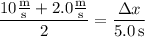Question

.A cart rolling down an incline for 5.0 seconds has an acceleration of 1.6 m/s2. If the cart has a beginning speed of 2.0 m/s, and its final velocity of 10 m/s, what was the car's displacement?

Answer 1

Use the formula,

`\Delta x=v_it+\frac12at^2`

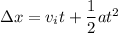
where
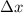`\Delta x` is the cart's displacement (from the origin),
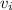`v_i` is its initial speed,
`a` is its acceleration, and
`t` is time.

`\Delta x=\left(2.0(\rm m)/(\rm s)\right)(5.0\,\mathrm s)+\frac12\left(1.6(\rm m)/(\mathrm s^2)\right)(5.0\,\mathrm s)^2`

`\implies\boxed{\Delta x=30\,\mathrm m}`

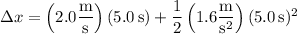
Alternatively, since acceleration is constant, we have

`\frac{v_f+v_i}2=\frac{\Delta x}t`

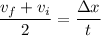
That is, we have these two equivalent expressions for average velocity, where
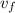`v_f` is the cart's final velocity. Solve for
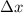`\Delta x`:

`\frac{10(\rm m)/(\rm s)+2.0(\rm m)/(\rm s)}2=(\Delta x)/(5.0\,\mathrm s)`

`\implies\boxed{\Delta x=30\,\mathrm m}`