Question :
Law of Cosines by ∆ ABC

Answer :

Law of Cosines by ∆ ABC:
Let three side be :

Formula:
- a² = b² + c² – 2bc (cos A)
- b² = a² + c² – 2ac (cos B)
- c² = b² + a² – 2ba (cos C)

Also known as :
Law of Cosines by ∆ ABC is also known as :

Define :
The law of cosines states that if any two sides(i.e a , b , or c) of a triangle and angle formed between them ( i.e ∠A , ∠B or ∠C) are give then we are able to find third side.
(side 1)² = (side 2)² + (side 3)² - 2 side 1 × side 2 (cos angle formed between side 2 and side 3)

Question :
Law of Sines by ∆ ABC

Answer :

Law of sines by ∆ ABC:
Let three side be :

Formula :
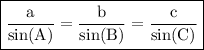

Define :
It is ratio between side of triangle (i.e a , b , c) and sin of angle formed (angle A , angle B or angle C) opposite to it. Above formula you can see side a is side angle and opposite angle sin i.e A are in ratio and they are equal to ratio of side b and sin of opposite angle to b i.e angle B.

Correct Question :
Explain SSA

Full form
S - Side
S - Side
A - Angle

Define :
This condition is seen when two triangles are congruent , when two sides are equal and angle which is not formed between them are equal.

Congruent triangles :
Congruent triangles are those triangles that have exact shape and size.

Conditions for congruent triangles :
Side - angle - side
angel - side - angle
side side angle
side side side