Question

A 4-pole, 3-phase induction motor operates from a supply whose frequency is 60 Hz. calculate: 1- the speed at which the magnetic field of the stator is rotating

Answer 1

The answer is below

Step-by-step explanation:

A 4-pole, 3-phase induction motor operates from a supply whose frequency is 60 Hz. calculate: 1- the speed at which the magnetic field of the stator is rotating. 2- the speed of the rotor when the slip is 0.05. 3- the frequency of the rotor currents when the slip is 0.04. 4- the frequency of the rotor currents at standstill.

Given that:

number of poles (p) = 4, frequency (f) = 60 Hz

1) The synchronous speed of the motor is the speed at which the magnetic field of the stator is rotating. It is given as:

`n_s=(120f)/(p)=(120*60)/(4)=1800\ rpm`

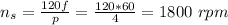
2) The slip (s) = 0.05

The speed of the motor (n) is the speed of the rotor, it is given as:

`n=n_s-sn_s\\\\n=1800-0.05(1800)\\\\n=1800-90\\\\n=1710\ rpm`

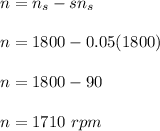
3) s = 0.04

The rotor frequency is the product of the supply frequency and slip it is given as:

`f_r=sf\\\\f_r=0.04*60\\\\f_r=2.4\ Hz`

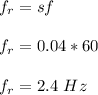
4) At standstill, the motor speed is zero hence the slip = 1:

`s=(n_s-n)/(n_s)\\ \\n=0\\\\s=(n_s-0)/(n_s)\\\\s=1`

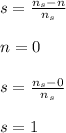
The rotor frequency is the product of the supply frequency and slip it is given as:

`f_r=sf\\\\f_r=1*60\\\\f_r=60\ Hz`