Question

If for two non-zero vectors a,b : ∣a+b∣=∣a−b∣ then angle between them is:______a. 0b. 45c. 60d. 90

Answer 1

`90^\circ`

Explanation:

Given two non zero vectors,
`\vec a, \vec b`.

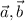Let the angle between the two vectors =
`\theta`

Given that:

`|\vec a+\vec b|=|\vec a-\vec b|`

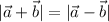
Let us have a look at the formula for magnitude of addition of two vectors:

`|\vec x+\vec y|=√(x^2+y^2+2xycos\theta)`

Where
`\theta` is the angle between the two vectors.

formula for magnitude of subtraction of two vectors:

`|\vec x-\vec y|=√(x^2+y^2-2xycos\theta)`

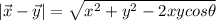
As per the given condition:

`√(a^2+b^2+2abcos\theta)=√(a^2+b^2-2abcos\theta)`

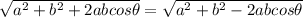
Squaring both sides:

`a^2+b^2+2abcos\theta=a^2+b^2-2abcos\theta\\\Rightarrow 2abcos\theta=-2abcos\theta\\\Rightarrow cos\theta = -cos\theta\\\Rightarrow 2cos\theta = 0\\\Rightarrow cos\theta = 0\\\Rightarrow \theta = 90^\circ`

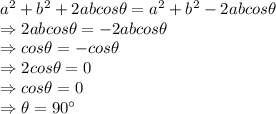
So, the angle between the two vectors is:
`90^\circ`