Answer:
The general solution of the equation is y =
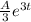 + 5
+ 5
Explanation:
Since the differential equation is given as y'(t) = 3y -5
The differential equation is re-written as
dy/dt = 3y - 5
separating the variables, we have
dy/(3y - 5) = dt
dy/(3y - 5) = dt
integrating both sides, we have
∫dy/(3y - 5) = ∫dt
∫3dy/[3(3y - 5)] = ∫dt
(1/3)∫3dy/(3y - 5) = ∫dt
(1/3)㏑(3y - 5) = t + C
㏑(3y - 5) = 3t + 3C
taking exponents of both sides, we have
exp[㏑(3y - 5)] = exp(3t + 3C)
3y - 5 =

3y - 5 =
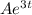
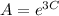
3y =
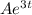 + 5
+ 5
dividing through by 3, we have
y =
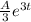 + 5
+ 5
So, the general solution of the equation is y =
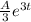 + 5
+ 5