Question

I put a picture and please solve

Answer 1

Answer: 12.25 or Approximately 12.3

Explanation:

To solve this problem, we first need to solve for x. We are given with 2ˣ=7.

To get x alone, we want to use natural log, or ln.

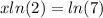`xln(2)=ln(7)` [once you find ln of both sides, you bring the x to the front]

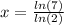`x=(ln(7))/(ln(2))` [divide both sides by ln(2)]

`x=2.81`

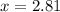
We now know that x is approximately 2.81. We can plug that in to find 4ˣ⁻¹.

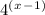`4^(^x^-^1^)` [plug in x]

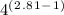`4^(^2^.^8^1^-^1^)` [parenthesis]

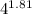`4^(1.81)` [exponent]

`12.3`

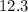
The answer is approximately 12.3. To get a more exact answer, you would plug in
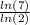`(ln(7))/(ln(2))` into x.

`4^{(ln(7))/(ln(2))-1 }`

`12.25`

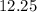
The exact answer would be 12.25. This is close to 12.3 since it is rounded up to one decimal place.